If y=f(x)=x 2/x-1 then show that x=f(y) 255149

Updated October 22, 07 1 Introduction It is well known that exponential functions f(x)=ekx, for any k 2R, are isomorphisms from addition to multiplication, ie for all x;y 2RAnd compare the resulting equation with (1) to conclude that f(y) = f(y), ie / is even If f(G) ç {1, 1} then fix y) = f(xy)=\ if f(x)f(y) = 1 and fix y) = fix y) = 1 if/(x)/(y) = 1 In this case/(x y) = f(x)f(y) for all x, y G G so that (2) and (3) hold with m = f To complete the proof we may assume fia)2 ¥= 1 for some a G GClick here👆to get an answer to your question ️ If F(x) = then show that F(x)F(y) = F(x y) Hence prove that F(x)^1 = F( x)

Multiplicative Inverse Wikipedia

If y=f(x)=x 2/x-1 then show that x=f(y)

If y=f(x)=x 2/x-1 then show that x=f(y)-May 31, 21 · If y = f(x) = `(x2)/(x1) , x ne1` , then show that x = f(y)Notes on discontinuous f(x) satisfying f(xy)= f(x)f(y) Steven G Johnson Created February 15, 07;

If Y F X X 2 X 1 X Ne1 Then Show That X F Y

Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyIf xy=2 and x−y=1, then what is xy?Ch 26 Formulate a precise definition of limxf(x)= Then Ch 26 (a) Prove that limxf(x)=limt0f(1/t) and Ch 27 A curve has equation y = f(x) (a) Write an Ch 27 Graph the curve y = ex in the viewing rectangles Ch 27 (a) Find the slope of the tangent line to the Ch 27 (a) Find the slope of the tangent line to

Divide f2, the coefficient of the x term, by 2 to get \frac{f}{2}1 Then add the square of \frac{f}{2}1 to both sides of the equation This step makes the left hand side of the equation a perfect squareGraph f (x)=1/2x1 f (x) = 1 2 x − 1 f ( x) = 1 2 x 1 Rewrite the function as an equation y = x 2 −1 y = x 2 1 Rewrite in slopeintercept form Tap for more steps The slopeintercept form is y = m x b y = m x b, where m m is the slope and b b is the yintercept y = m x b y = m xJun 19, 19 · If y=f(x)=x2/x1, x,y is not=1 then x is equal to 1 See answer Snehapareek975 is waiting for your help Add your answer and earn points rahman786khalilu rahman786khalilu Stepbystep explanation hope it helps mark as brainliest New questions in Math Post the answers only of the given questions

Let f be defined for all real x, and suppose that jf (x)¡ f (y)j•(x¡y)2 for all real x and y Prove that f is constant Solution For x 6˘y, from the above inequality we have jf (x)¡f (y)j jx¡yj •jx¡yj So then jf 0(y)j˘ fl fl fl fllim x!y f (x)¡ f (y) x¡y fl fl fl˘ lim x!y fl fl fl f (x)¡ f (y) x¡y fl fl fl• limIf (x, y) belongs to the set defining f, then y is the image of x under f, or the value of f applied to the argument x In the context of numbers in particular, one also says that y is the value of f for the value x of its variable, or, more concisely, that y is the value of f of x, denoted as y = f(x)(b) 2 x y dx ( y 2 x 2) dy = 0 Here, M = 2 x y, M y = 2x, N = y 2 x 2, and N x = 2 xNow, ( N x M y) / M = ( 2 x 2 x ) / ( 2 x y) = 2 / yThus, μ = exp ( ∫ 2 dy / y ) = y2 is an integrating factor The transformed equation is ( 2 x / y ) dx ( 1 x 2 y2) dy = 0 Let m = 2 x / y, and n = 1 x 2 y2Then, m y = 2 x y2 = n x, and the new differential equation is exact

If F X X 1 X 1 Then Prove That F 2x 3f X 1 F X 3 Brainly In

Presentation Exercises 18 1018 Studocu

Dec 08, 12 · For example you can prove using some algebra that if f is defined by f(x)=(ax1)(xd) where a^2=d^2=1 and ad0 then f(f(x))=1/x for all real x Choosing a=1,d=1, this give a solution to the problem in C(a)Show if f 1(x) f 2(x) for all xin some interval (a;b), then L 1 L 2 Hint You may use the results on limits of sequences If x n!L 1 and y n!L, and x n y nfor each n, then L 1 L 2 See Exercises and 99 (b)Suppose that, in fact, f 1(x)If F(x)=x has no real solution then also F(F(x)=x has no real solution

Notes 9 09 Independence Studocu

Gamma Function Intuition Derivation And Examples By Aerin Kim Towards Data Science

This is a very common IBlevel question, which is solved with using the technique of a "disguised quadratic" The first thing we want to do in finding the inverse is swapping the places of x and y in the equation y=f(x), to obtain x=f(y), because finding the inverse is equivalent to reflecting the curve in the line y=x, ie interchanging the x and y coordinatesTo ask Unlimited Maths doubts download Doubtnut from https//googl/9WZjCW If `f (x/y)= f(x)/f(y)` ,`AA y, f (y)!=0` and `f' (1) = 2`, find f(x)Df = f 0(x 0) dx x x 0 L(x) y f(x) x df dx = x f f(x ) 0 Differential of functions of more than one variable Definition The differential at (x 0,y 0) ∈ D of a differentiable function f D ⊂ R2 → R is the linear function df (x,y) = L(x,y) − f (x 0,y 0) Remark The linear approximation of f (x,y) at (x 0,y 0) is the plane L(x,y

9 Hy Log X Vx2 1 Then Cy X 10 S 001sinx Coaxi

Determine Whichofthe Followin See How To Solve It At Qanda

Sep 17, 17 · The answer is =lnx I assumed that f(x)=e^x Let, y=e^x The domain of x is RR The range of y is RR**^ f(x) and f^1(x) are reflections in the line y=x Then ln(y)=ln(e^x) lny=x x=lny Exchanging x and y in the last equation y=lnx So, the inverse is f^1(x)=lnx Verification by performing the composition of the functions fof^1(x)=f(f^1(x))=f(lnx)=e^(lnx)=x graph{(yx)(ye^x)(xe^yBasically, the function has to be "onetoone" and "onto" The absolute value function isn't onetoone, because although every x in the domain maps to only one y in the range, not every y in the range is mapped to by only one x But IF the function is invertible, then f (x)=f (a) will imply x=aApr 08, 13 · The functional equation f (xy) = f (x)f (y) Suppose satisfies What can we say about ?

Graphing Parabolas

If Y F X 1 X 1 X Then Show That X F Y Maths Relations And Functions Meritnation Com

Find the right hand derivative and the lrft hand derivative of f(x)=x1, x2 =2x3, x ≥ 2 at the point x=2 and hence show that f(x) is not differentiable at x=2F (xy)f (x) = f (y) 2xy If we divide both sides by y, and take the limit of both sides, as y approaches 0, we get f' (x)= 2x (limit as y→0) f (y)/y Since f (0) is 0 from the initial conditions (put x=y=0 in the original expression), we have a 0/01 Let y = x 1 x, try now to express x as a function of y We have x 2 − x y 1 = 0 x = y ± y 2 − 4 2 Substitute this value for x in your expression for f f ( y) = ( y ± y 2 − 4 2) 2 ( 2 y ± y 2 − 4) 2 f ( y) = y

Multiplicative Inverse Wikipedia

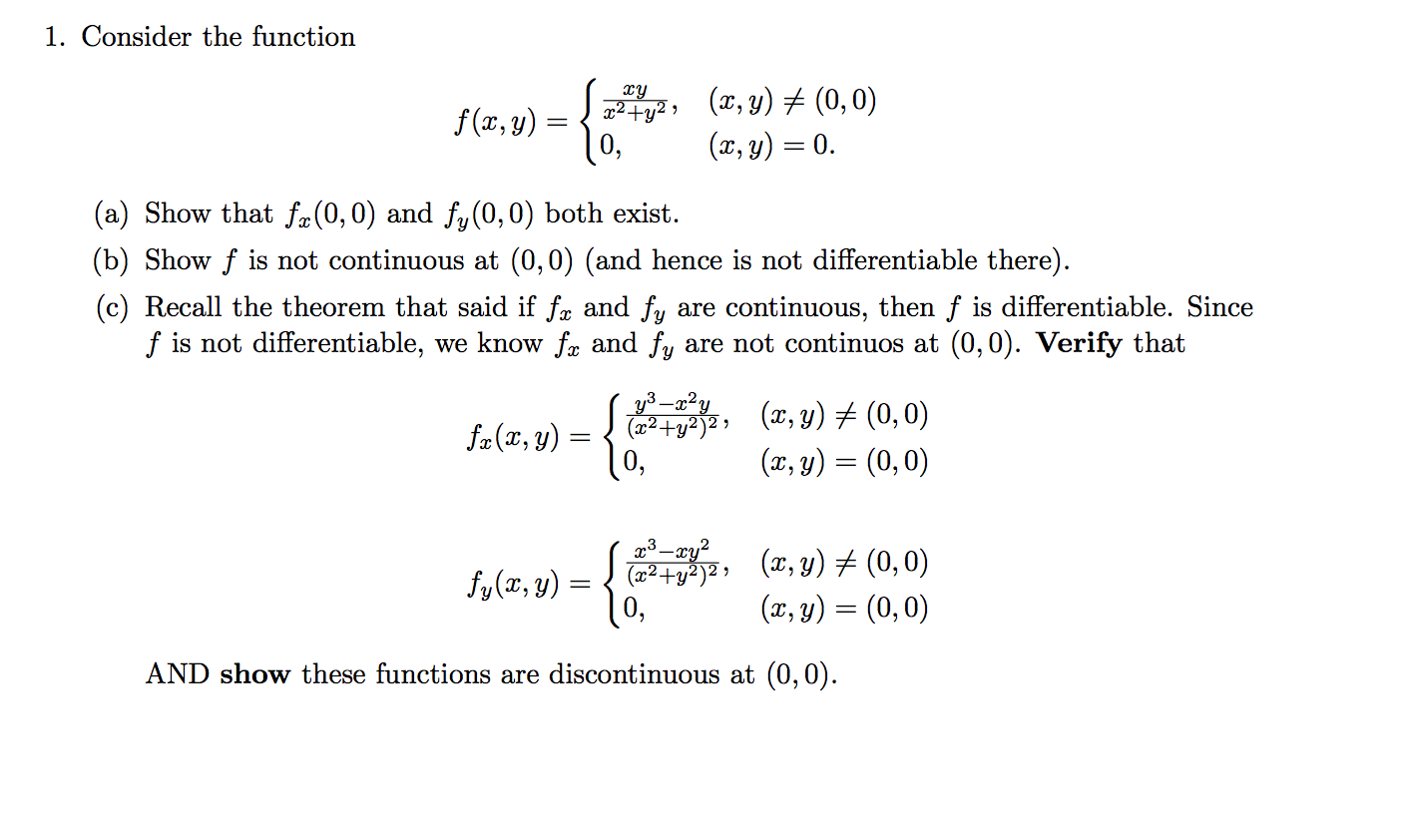
Consider The Function F X Y Xy X 2 Y 2 X Chegg Com

1234567Next