Find the derivative of the function f(x) = x x 5 using the limit de nition of the derivative Note the format of the solution below It is important to carry the limits and show all calculations in order to recieve full credit f0(x) = lim h!0 f(x h) f(x) h = lim h!0 xh xh 5 x x 5 h = lim h!0 (x h)(x 5) x(x hThe square function given by f(x) = x2 is differentiable at x = 3, and its derivative there is 6 This result is established by calculating the limit as h approaches zero of the difference quotient of f(3)Business Finance Derivative (finance) Use f' (x) = limit as h approaches 0 of (f (x h) f (x))/ (h) to find the derivative at x for the
Ii Deterrmine The Derivatives Of The Functions Us Gauthmath
What does h mean in derivative formula
What does h mean in derivative formula-Transcribed image text For the function f(x)=x²3x1 a) Find the derivative f'(x) by using the definition f'(x) = lim f(xh)f(x) h 10 (xh) 2 3(xh)(x23x1) h tha2xh 3x 3h2 30 4 h hat 3x2xK h 2x3 b) Find the slope of the tangent line to y = f(x) at the point where x = 5 c) Write the equation of the tangent line to y = f(x) at the point (5,11)• Again since the function is approximated by the interpolating function , the second derivative at node x 1 is approximated as • Substituting in for the expression for x 1 = h g 2 x 1 g = 2 h g 2 x 1 f 2 – 2f 1 f o h2 = fx gx f 2 x 1 g 2 x 1 g 2 x 1 f 2 x 1 f 2 – 2f 1 f o h2
Solutions Manual For Calculus For Business Economics Life Sciences And Social Sciences 13th Edition By Sullivan8 Issuu
• Constant Multiple Rule g(x)=c·f(x)theng0(x)=c·f0(x) • Power Rule f(x)=x n thenf 0 (x)=nx n−1 • Sum and Difference Rule h(x)=f(x)±g(x)thenh 0 (x)=f 0 (x)±g 0 (x)Feb 11, 19 · For the function f, its derivative is said to be f'(x) given the equation above exists Check out all the derivative formulas here related to trigonometric functions, inverse functions, hyperbolic functions, etc Properties of Derivatives Some of the important properties of derivatives are given belowDon't try to learn a formula like this You should know the product rule for two functions Well, mathf(x)/math is one function and mathk(x)=g(x)h(x)/math is another So the derivat
Nov 10, · The limit definition of the derivative, \(f'(x)=lim_{h\to 0} \frac{f(xh)f(x)}{h}\), produces a value for each \(x\) at which the derivative is defined, and this leads to a new function whose formula is \(y=f'(x)\) Hence we talk both about a given function \(f\) and its derivative \(fA as a variable, we may define a function called the derivative function In terms of limits, the derivative function of a function f , denoted by f ′ is given by f ′ ( x) = lim h → 0 f ( x h) − f ( x) h Using limit laws, we can show that differentiable functions are continuousFinding the Derivative of a Quadratic Function Find the derivative of the function f(x) = x2 − 2x Solution Follow the same procedure here, but without having to multiply by the conjugate f ′ (x) = lim h → 0 ( ( x h) 2 − 2 ( x h)) − ( x2 − 2x) h Substitutef(x h) = (x h)2 − 2(x h)and f(x) = x2 − 2xinto f
Steps Solve for the inner derivative of g ( x) = x 2 d g d x = 2 x Solve for the outer derivative of h ( x) = x 3, using a placeholder b to represent the inner function x 2 d h d b = 3 b 2 Swap out the placeholder variable (b) for the inner function (g (x)) 3 ( x 2) 2 3 x 4For a function f (x), the difference quotient would be f (xh) f (x) / h, where h is the point difference and f (xh) f (x) is the function difference The difference quotient formula helps to determine the slope for the curved lines The f (xh) f (x) / h calculator can be used to find the slope value, when working with curved linesF (x h) − f (x) in such a way that we can divide it by h To sum up The derivative is a function a rule that assigns to each value of x the slope of the tangent line at the point (x, f (x)) on the graph of f (x) It is the rate of change of f (x) at that point
Calculus Index Cards
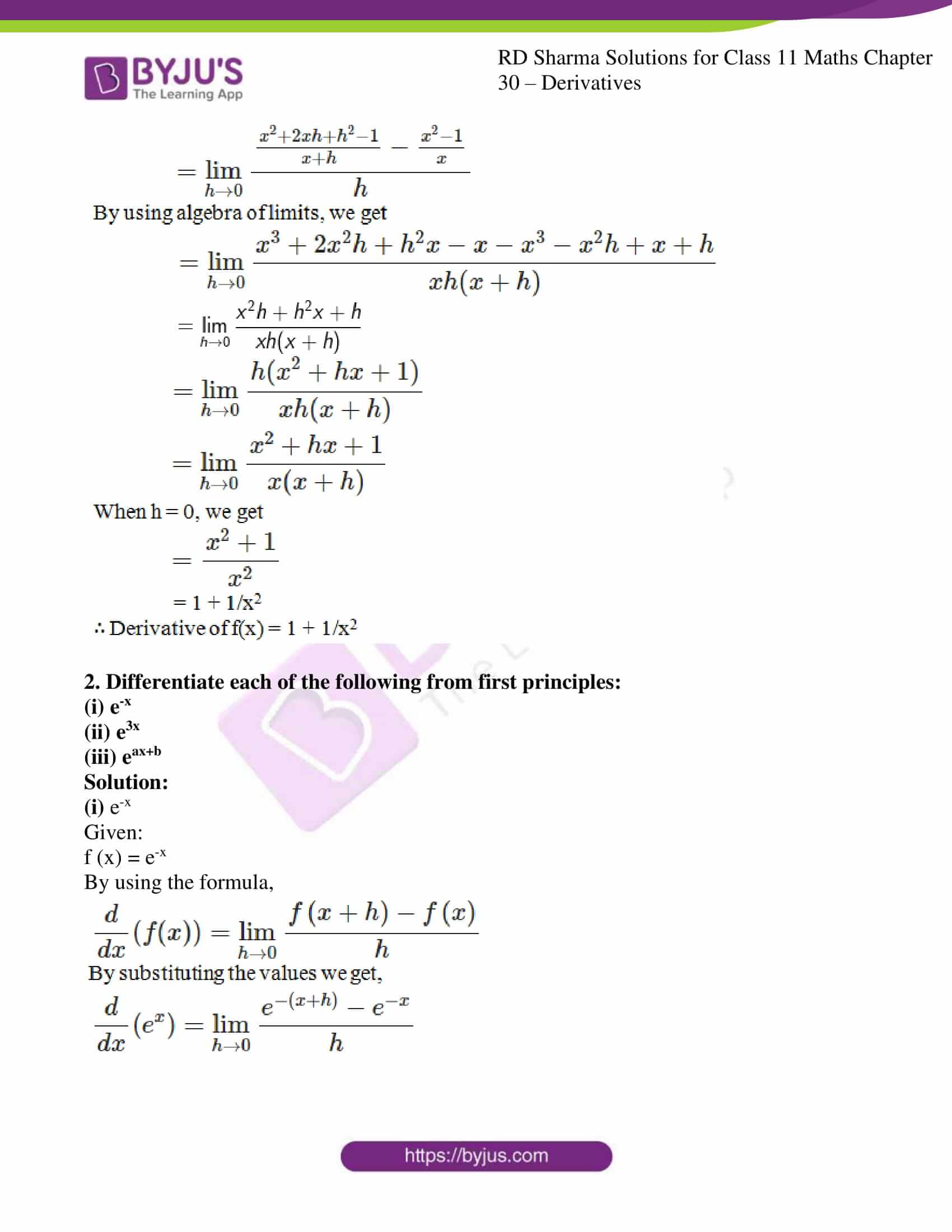
Rd Sharma Solutions For Class 11 Chapter 30 Derivatives Download Free Pdf Available
Interactive graphs/plots help visualize and better understand the functionsSolution We can use the formula for the derivate of function that is the sum of functions f(x) = f 1 (x) f 2 (x), f 1 (x) = 10x, f 2 (x) = 4y for the function f 2 (x) = 4y, y is a constant because the argument of f 2 (x) is x so f' 2 (x) = (4y)' = 0 Therefore, the derivative function of f(x) is f'(xSuppose h ( x) = f ( x) g ( x), where f and g are differentiable functions and g ( x) ≠ 0 for all x in the domain of f Then The derivative of h ( x) is given by g ( x) f ′ ( x) − f ( x) g ′ ( x) ( g ( x)) 2 "The top times the derivative of the bottom minus the bottom times the derivative of the top, all over the bottom squared
Solutions Manual For Calculus For Business Economics Life Sciences And Social Sciences 13th Edition By Sullivan8 Issuu
Mathtype Twitter પર Usually When Deriving A Function More Than Once One Just Derives And Then Derives Again And So On Alternatively There Is This Nice Formula Involving Pascal S Triangle To Calculate
Visual Calculus Definition of derivative Definition of Derivative Definition Let y = f (x) be a function The derivative of f is the function whose value at x is the limit provided this limit exists If this limit exists for each x in an open interval I, then we say that f is differentiable on I ExamplesH(x) = f(x)±g(x) then h′(x) = f ′(x) ± g′(x) The difference and sum rule will make sure the derivative of sum of function is the sum of their derivatives calculated by differentiation calculatorFor secondorder derivatives, it's common to use the notation f"(x) For any point where x = a, the derivative of this is f'(a) = lim(h→0) f(ah) f(h) / h The limit for this derivative may not exist If there is a limit, then f (x) will be differentiable at x = a The function of f'(a) will be the slope of the tangent line at x=a
Second Derivative Wikipedia
5 Numerical Differentiation Pdf Free Download
Dec 01, · Definition of the Derivative The derivative of f(x) is mostly denoted by f'(x) or df/dx, and it is defined as follows f'(x) = lim (f(xh) f(x))/h With the limit being the limit for h goes to 0 Finding the derivative of a function is called differentiationProblems involving derivatives 1) f(x) = 10x 4y, What is the first derivative f'(x) = ?F(xh)−f(x) h − h 2 f00(ξ), ξ ∈ (x,xh) (53) Since this approximation of the derivative at x is based on the values of the function at x and x h, the approximation (51) is called a forward differencing or onesided differencing The approximation of the derivative at x that is based on the values of the function at x−h and x, ie, f0(x) ≈
Example 6 Find Derivative Of F X 2x2 3x 5 At X 1
5 Numerical Differentiation Pdf Free Download
Derivative Here, n = 4 d/dx x(n) = nx n1 = d/dx (xThe first one is used to evaluate the derivative in the point x = a That is \lim_{x\to a} \frac{f(x) f(a)}{xa} = f'(a) The second is used to evaluate the derivative for all x That is \lim_{h\to 0} \frac{f(xh) f(x)}{h} = f'(x)The derivative at a point The derivative of a function f(x) at a point (a, f(a)) is written as f ′ (a) and is defined as a limit f ′ (a) = lim h → 0f(a h) − f(a) h
Marthamatics Differentiation From First Principles Light
Solved Approximating Derivatives Forward Difference F X Chegg Com
We know that if `f` is a function, then for an `x`value `c` `f'(c)` is the derivative of `f` at `x = c` `f'(c)` is slope of the line tangent to the `f`graph at `x = c` `f'(c)` is the instantaneous rate of change of `f` at `x = c` In this applet we move from thinking about the derivative of `f` at a point, to thinking about the derivativeDerivative The derivative of a function f (x) is another function denoted or f ' (x) that measures the relative change of f (x) with respect to an infinitesimal change in x If we start at x = a and move x a little bit to the right or left, the change in inputs is ∆x = x a, which causes a change in outputs ∆x = f (x) f (a)Nov 25, 17 · So we use different polynomials f(x) = 1, f(x) = (x − x0) / h, f(x) = ((x − x0) / h)2, f(x) = ((x − x0) / h)3 and f(x) = ((x − x0) / h)4 (1) A B C D E = 0 (2) − A C 2D 3E = 1 / h (3) A C 4D 9E = 2 / h2 (4) − A C 8D 27E = 3 / h3 (5) A C 16D 81E = 4 / h4
Numerical Differentiation Wikiwand
Derivative Of 2x 2 3x 4 Math Calculus Showme
In calculus, the quotient rule is a method of finding the derivative of a function that is the ratio of two differentiable functions Let f(x)=g(x)/h(x), where both g and h are differentiable and h(x)≠0These are called higherorder derivatives Note for secondorder derivatives, the notation f ′′(x) f ″ ( x) is often used At a point x = a x = a, the derivative is defined to be f ′(a) = lim h→0 f(ah)−f(h) h f ′ ( a) = lim h → 0 f ( a h) − f ( h) hDerivative by first principle refers to using algebra to find a general expression for the slope of a curve It is also known as the delta method The derivative is a measure of the instantaneous rate of change, which is equal to f ′ ( x) = lim h → 0 f ( x h) − f ( x) h f' (x) = \lim_ {h \rightarrow 0 } \frac { f (xh) f (x
Differentiation The Numerical Methods Guy
Basics Of Derivatives
Therefore as h goes to 0, an approximation of a value that is O(hp) gets closer to the true value faster than one that is O(hq) By computing the Taylor series around a = xj at x = xj − 1 and again solving for f′(xj), we get the backward difference formula f′(xj) ≈ f(xj) −The Derivative Calculator supports computing first, second, , fifth derivatives as well as differentiating functions with many variables (partial derivatives), implicit differentiation and calculating roots/zeros You can also check your answers!0 to find the derivative, it is not supposed to matter whether h is positive or negative lim h!0 f(x h) ¡ f(x) h = lim h!0¡ f(x h) ¡ f(x) h For a function of a complex variable f(z), when we find the derivative by letting ∆z !
Find The Derivative Of A Function At A Certain Num Gauthmath
Ii Deterrmine The Derivatives Of The Functions Us Gauthmath
0, the direction of ∆z is not supposed to matter However, since ∆z is a vector in two dimensions, there are now many more directionsWhat is the formula for the derivative of f(x) g(x) h(x)?This tutorial is well understood if used with the difference quotient The derivative f ' of function f is defined as f' (x) = \lim_ {h\to\ 0} \dfrac {f (xh)f (x)} {h} when this limit exists Hence, to find the derivative from its definition, we need to find the limit of the difference quotient as h
Solved Find The Difference Quotient Off Thatis Find F X H F X H H Not Equals 0 H0 For The Following Function Be Sure To Simplify Course Hero
Basics Of Derivatives
The product rule is a formula that is used to determine the derivative of a product of functions There are a few different ways that the product rule can be represented Below is one of them Given the product of two functions, f(x)g(x), the derivative of the product of those two functions can be denoted as (f(x)·g(x))'Transcribed image text Assume that f(x) is a differentiable function for all z Find the derivative of each of the functions h(x) For the purpose of this exercise, use f for f(x) and F for f'(x) h(x) = r* f() h'(x) Preview Assume that f(x) and g(2) are both differentiable functions for allCentered Difference Formula for the First Derivative We want to derive a formula that can be used to compute the first derivative of a function at any given point Our interest here is to obtain the socalled centered difference formula We start with the Taylor expansion of the function about the point of interest, x, f(x±h) ≈ f(x)±f0(x
Calculus Review Derivative Asu
Finding A Derivative Using The Definition Of A Derivative Youtube
Find the derivative of the following function {eq}h(x) = 3^{2x 4} {/eq} Applying the Rules of Differentiation We can find the derivative of an exponential function by applying the standardFind the derivatives of cos x, tan x, cot x, sec x and csc x using their relations to sin x Use the facts that x1/n is the inverse function to xn to find (x1/n)' and also find the derivatives of the inverse functions to exp (x), sin x, and tan x (namely ln (x), arcsin x, and arctan x) by applying the "inverse rule" just describedOct 07, 15 · Let f (x) = √1 2x Then the derivative at x = a is defined as the following limit f '(a) = lim h→0 f (a h) −f (a) h = lim h→0 √1 2(a h) − √1 2a h
Numerical Differentiation Pages 1 5 Flip Pdf Download Fliphtml5
B Deterrmine The Derivatives Of The Functions U Gauthmath
Defined, respectively, in terms of the functional values f(x− h) and f(x), and f(x− h) and f(xh) Twopoint Forward Difference Formula (FDF) f′(x) ≈ f(xh) −f(x) h (73) Twopoint Backward Difference Formula (BDF) f′(x) ≈ f(x) −f(x−h) h (74) Twopoint Central Difference Formula (CDF) f′(x) ≈ f(xh) −f(x) hMake use of this free online derivative calculator to differentiate a function You can use this derivative calculator to convert functions from one form to another Example What is the derivative of x 4?Jan 02, 21 · Let f be a function The derivative function, denoted by f ′, is the function whose domain consists of those values of x such that the following limit exists f ′ (x) = lim h → 0f(x h) − f(x) h A function f(x) is said to be differentiable at a if f ′ (a) exists
Calculating A Derivative Using The Definition Of A Derivative Example 1 Youtube
5 Numerical Differentiation Pdf Free Download
When we take h !The derivative of a function f at the point x is defined as the limit of a difference quotient f0(x) = lim h→0 f(xh)−f(x) h In other words, the difference quotient f(xh)−f(x) h is an approximation of the derivative f0(x), and this approximation gets better as h gets smaller How does the error of the approximation depend on h?1 Solved example of definition of derivative d e r i v d e f ( x 2) derivdef\left (x^2\right) derivdef (x2) 2 Apply the definition of the derivative f ′ ( x) = lim h → 0 f ( x h) − f ( x) h \displaystyle f' (x)=\lim_ {h\to0}\frac {f (xh)f (x)} {h} f ′(x)= h→0lim
Calculus I Derivative Using Definition F X 3x 2 5x Youtube
The Formula F X F X H F X H 2h Can Be Chegg Com
Free derivative calculator differentiate functions with all the steps Type in any function derivative to get the solution, steps and graph
Taylor Series In Numerical Differentiation Mathematics Stack Exchange
Answered Find The Actual Error When The First Bartleby
The Derivative
Calculus For Business Economics And The Social And Life Scienc Brief Edition 11th Edition By Hoffman By Jaqis162 Issuu
Finding The Derivative Of F X X 2 X 3 By Using The Definition Youtube
Derivatives Of Inverse Functions From Equation Video Khan Academy
4 Questions On Calculus I Midterm Exam Math 1241 Docsity
Finite Differences Aim Of This Section Finite Difference Derivative
Ppt Numerical Differentiation Powerpoint Presentation Free Download Id 5987
Basic Derivative Rules Table Video Khan Academy
Ex 13 2 10 Find Derivative Of Cos X From First Principle Teachoo
5 Numerical Differentiation Pdf Free Download
Definition Of Derivative Defined Illustrated W Examples
Worked Example Derivative From Limit Expression Video Khan Academy
Slope Of The Secant Line Formula Learn The Formula To Find The Slope Of Secant Line
Prove That Derivative Of Tan X Is Sec 2 X By First Principle
Prove That Derivative Of Tan X Is Sec 2 X By First Principle
3 In Class We Discussed The Central Difference Chegg Com
Let The Derivative Of F X Be Defined As D F X Lim H 0 F 2 X
Numerical Differentiation Ppt Download
Ex 13 2 4 Find Derivative From First Principle I X3 27
Solutions Manual For Calculus For Business Economics And The Social And Life Scienc Brief Edition 11 By Marke Issuu
Derivative Formulas Studocu
Using The Formula Fprimex Lim Text A H Arrow Gauthmath
Calculus The Definition Of The Derivative
How To Find The Difference Quotient F X H F X H For F X 1 X Youtube
Difference Quotient Youtube
5 Numerical Differentiation Pdf Free Download
Basics Of Derivatives
Numerical Methods Some Example Applications In C Ppt Download
Answered V0 Fu 23 The Derivative Find The Bartleby
Derivative Wikipedia
Computational Methods 18 19 Fall Chapter 6 Ppt Download
Differentiation Of Exponential Functions Ppt Download
L Hopital S Rule Wikipedia
Question Video Using The Product Rule Nagwa
Ii Directions Find The Derivative Of The Followi Gauthmath
Calculus The Derivative And The Applications Of Differentiation Ppt Download
Numerical Differentiation Wikipedia
Derivative As A Function Docsity
Calculus Index Cards
How To Compute The Difference Quotient F X H F X H Youtube
Dilrections Find The Derivative Of A Tunction At Gauthmath
Solutions To Odd Numbered Problems From Chapter 3 Of
The Quotient Rule For Differentiation Math Insight
Derivatives What Nancypi Bettergradesfast Tv
Differentiation The Numerical Methods Guy
The Four Step Process For F X X 2 8x 2 Youtube
2 Limits And Derivatives Pdf Free Download
Numerical Differentiation Ppt Download
Solved By Calculating The Second Derivative Using The For Chegg Com
Solved By Calculating The Second Derivative Using The For Chegg Com
What Is Derivative Formula
Differentiation The Numerical Methods Guy
Numerical Differentiation Forward Difference Formula Ppt Download
Instantaneous Rate Of Change Formula By Tutorvista Team Issuu
Solved Question 1 5 Marks Consider The Function F X 4x 3x2 Find The Derivative Of F X Using The Limit Definition Determine An Equation Of Course Hero
Name Group Members Exploration 4 2a Derivative Of A Pages 1 12 Flip Pdf Download Fliphtml5
0 件のコメント:
コメントを投稿